Poker Probability and Odds: The Math Behind the Game

Poker probability involves mathematical analysis of different events’ likelihood in a Poker game. This subject can be complex, but it’s essential for any player aiming for long-term success.

Your chances of winning a Poker hand rely on the cards you have and the ones already on the table. You can make better game decisions by knowing the odds of different Poker hands.

In this section, you will learn about the poker variant and hand probabilities that offer the best chance of winning. You will also understand the difference between odds and probabilities.

Poker Hand Probabilities

In a standard 52-card deck, there are as many as 2,598,960 possible ways to select five cards.

Of all the poker hands, getting a royal flush is the most difficult hand to obtain (with odds of 1 in 649,739), while drawing a high card is the easiest hand to get (with odds of 1 in 2).

POKER HAND
DISTINCT HANDS	FREQUENCY	PROBABILITY	ODDS
High Card	1,302,540	50.12%	1 in 2
One Pair	1,098,240	42.26%	1 in 2.4
Two Pair	123,552	4.75%	1 in 21
3-of-a-Kind	54,912	2.11%	1 in 47
Straight Hand	10,200	0.39%	1 in 255
Flush Hand	5,108	0.20%	1 in 509
Full House Hand	3,744	0.14%	1 in 694
4-of-a-Kind	624	0.024%	1 in 4,165
Straight Flush	36	0.0014%	1 in 72,193
Royal Flush	4	0.00015%	1 in 649,740

Although getting these strong hands is difficult, skilled Poker players can enhance their winning chances by understanding the odds and making wise decisions.

This section explores the probabilities of various Poker hands and delves into pre-flop and post-flop scenarios.

Probability of High Card Hand

The “High Card” hand is the simplest and weakest hand possible.

It occurs when a player’s five cards do not form unique combinations like pairs, straights, or flushes. Instead, the hand’s ranking is determined solely by the highest card in it.

Calculating the Probability of a High Card:

To calculate the probability of getting a high card hand, consider the total number of possible 5-card combinations from a standard deck of 52 cards, known as the “sample space.”

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”

Now, to find the probability of a high card hand, subtract the number of other possible Poker hands from the total number of 5-card combinations and divide by the total number of combinations:

• Probability of a High Card Hand with 5-card,

= [C(52, 5) – (Number of Other Hands)] / C(52, 5)

Calculating this probability involves some complex math, but it’s approximately 50.1177%. In a standard 5-card Poker game, you have a 50.1177% chance of ending up with a high card hand.

Probability of One-Pair Hand

A “One Pair” hand is a step up from a “High Card” hand, and it’s the first rank on the Poker hand hierarchy that involves forming a specific combination.

In a One Pair hand, a player has two cards of the same rank and three unrelated cards. 

Calculating the Probability of a One-Pair Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”

To calculate the number of One Pair hands, we can use the following formula:

• Number of One Pair Hands:

 = C(13, 1) * C(12, 3) * C(4, 2) * C(4, 1)3

• C(13, 1): Choose one rank out of 13 available ranks for the pair.
• C(4, 2): Choose 2 suits out of 4 for the pair.
• C(12, 3): Choose 3 ranks out of the remaining 12 for the other three cards.
• C(4, 1)3: Choose 1 suit for each of the three unrelated cards.

Now, let’s calculate the probability:

• Probability of a One-Pair Hand in 5-Card Poker:

= (Number of One Pair Hands) / (Total 5-card combinations)

= [C(13, 1) * C(12, 3)* C(4, 2) * C(4, 1)3] / C(52, 5)

Precisely calculating this probability reveals 42.26%, meaning that in a standard 5-card Poker game, you have a 42.26% chance of ending up with a One-Pair Hand.

Probability of Two-Pair Hand

A “Two Pair” hand represents a more favorable hand ranking than a  “One Pair.” It occurs when a player has two sets of pairs with matching ranks and an unrelated fifth card.

Calculating the Probability of a Two-Pair Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”

To calculate the number of Two-Pair hands, we use the following formula:

• Number of Two-Pair Hands,

= C(13, 2) * C(4, 2)2 * C(4, 1)* C(11, 1)

• C(13, 2): Choose two ranks out of the 13 available ranks for the pairs.
• C(4, 2)2: Choose 2 suits out of 4 for each pair.
• C(11, 1): Choose 1 rank from the remaining 11 for the fifth card.
• C(4, 1): Choose 1 suit for the fifth card.

Now, let’s calculate the probability of getting a Two-Pair hand:

• Probability of a Two-Pair Hand in 5-card Poker:

= (Number of Two-Pair Hands) / (Total 5-card combinations)

= [C(13, 2) * C(4, 2)2 * C(4, 1)* C(11, 1)] / C(52, 5)

The probability, through manual calculation, is approximately 4.75%. In a standard 5-card Poker game, you have a 4.75% chance of being dealt or forming a Two-Pair hand.

Probability of Three-of-a-Kind Hand

A “Three-of-a-Kind” hand is a moderately strong hand involving three cards of the same rank and two unrelated cards that do not form any other unique combination. 

Calculating the Probability of a Three-of-a-Kind Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”

To calculate the number of Three-of-a-Kind hands, we use the following formula:

• Number of Three-of-a-Kind Hands:

= C(13, 1) * C(12, 2)* C(4, 3) * C(4, 1)2

• C(13, 1): Choose one rank from the 13 available ranks for the Three-of-a-Kind.
• C(4, 3): Choose 3 suits out of 4 for the Three-of-a-Kind.
• C(12, 2): Choose 2 ranks out of the remaining 12 for the two unrelated cards.
• C(4, 1)2: Choose 1 suit for each of the two unrelated cards.

Now, let’s calculate the probability of getting a Three-of-a-Kind hand:

• Probability of a Three-of-a-Kind Hand in 5-Card Poker:

= (Number of Three-of-a-Kind Hands) / (Total 5-card combinations)

= [C(13, 1) * C(12, 2)* C(4, 3) * C(4, 1)2] / C(52, 5)

While somewhat complex to calculate manually, the probability is approximately 2.11%. In a standard 5-card Poker game, you have about a 2.11% chance of being dealt or forming a Three-of-a-Kind hand.

Probability of Straight Hand

A “Straight” hand in Poker is a strong hand comprising five consecutive cards of any suit. 

Calculating the Probability of a Straight Hand:

A Straight hand forms when five cards are arranged sequentially, like 5-6-7-8-9 or 10-J-Q-K-A. like 5-6-7-8-9 or 10-J-Q-K-A. The suits of the cards can be mixed, meaning they don’t have to be of the same suit.

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”
• The number of Straight Hands:
• There are 10 possible sequences of 5 consecutive cards, each with 4 suits.

Now, let’s calculate the probability of getting a Straight hand:

• Probability of a Straight Hand in 5-Card Poker:

= (Number of Straight Hands) / (Total 5-card combinations)

= (10 * 45) / C(52, 5)

Although it is not as straightforward as others, this probability is approximately 0.39%. In a standard 5-card Poker game, you have about a 0.39% chance of being dealt or forming a Straight hand.

Probability of Flush Hand

In Poker, a “Flush” hand is a moderately strong hand that consists of five cards of the same suit, but they do not need to be in sequential order. 

Calculating the Probability of a Flush Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”

To calculate the number of Flush hands, consider how to pick five cards all of the same suit. Then, subtract the straight flush options (since we’ve already counted them as straight).

• Number of Flush Hands:

Number of Flush Hands = C(13, 5) * 4 – Number of Straight Flush Hands

• Number of Straight Flush Hands: This is the number of straight flushes (which counted as Straights). 

There are 10 possible sequences of 5 consecutive cards, each with 4 suits.

Now, let’s calculate the probability of getting a Flush hand:

• Probability of a Flush Hand in 5-Card Poker:

= (Number of Flush Hands) / (Total 5-card combinations)

= [(C(13, 5) * 4) – (10 * 4)] / C(52, 5)

This probability, though involving some calculations, is approximately 0.20%. In a standard 5-card Poker game, you have a 0.20% chance of being dealt or forming a Flush hand.

Probability of Full House Hand

A “Full House” hand consists of three cards of one rank and two cards of another. Players consider Full House to be a strong hand.

Calculating the Probability of a Straight Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”

To calculate the number of Full House hands, we use the following formula:

• Number of Full House Hands,

= C(13, 1) * C(12, 1)* C(4, 3) * C(4, 2)

• C(13, 1): Choose one rank from the 13 available ranks for the three-of-a-kind.
• C(4, 3): Choose 3 suits out of 4 for the three-of-a-kind.
• C(12, 1): Choose one rank out of the remaining 12 for the pair.
• C(4, 2): Choose 2 suits out of 4 for the pair.

Now, let’s calculate the probability of getting a Full House hand:

• Probability of a Full House Hand in 5-Card Poker:

= (Number of Full House Hands) / (Total 5-card combinations)

= [C(13, 1) * C(12, 1)* C(4, 3) * C(4, 2)] / C(52, 5)

The calculations resulted in a probability of approximately 0.14%. In a standard 5-card Poker game, you have about a 0.14% chance of being dealt or forming a Full House hand.

Probability of Four-of-a-Kind Hand

In Poker, a “Four-of-a-Kind” hand is a powerful hand consisting of four cards of the same rank and one unrelated card.

Calculating the Probability of a Flush Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”

To calculate the number of Four-of-a-Kind hands, we use the following formula:

• Number of Four-of-a-Kind Hands,

= C(13, 1) * C(12, 1) * C(4, 4) * C(4, 1)

• C(13, 1): Choose one rank from the 13 available ranks for the four-of-a-kind.
• C(4, 4): Choose all 4 suits for the four-of-a-kind.
• C(12, 1): Choose one rank out of the remaining 12 for the unrelated card.
• C(4, 1): Choose 1 suit for the unrelated card.

Now, let’s calculate the probability of getting a Four-of-a-Kind hand:

• Probability of a Four-of-a-Kind Hand in 5-Card Poker:

= (Number of Four-of-a-Kind Hands) / (Total 5-card combinations)

= [C(13, 1) * C(12, 1) * C(4, 4) * C(4, 1)] / C(52, 5)

The probability through manual calculation comes up to approximately 0.024%. In a standard 5-card Poker game, you have about a 0.024% chance of being dealt or forming a Four-of-a-Kind hand.

Probability of Straight Flush Hand

A “Straight Flush” hand is one of the strongest hands possible. It occurs when a player has five consecutive cards of the same suit. 

Calculating the Probability of a Flush Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”
• Number of Straight Flush Hands:
• There are 10 possible sequences of 5 consecutive cards, each with 4 suits.

Now, let’s calculate the probability of getting a Straight Flush hand:

• Probability of a Straight Flush Hand in 5-Card Poker:

= (Number of Straight Flush Hands) / (Total 5-card combinations)

= (10 * 4) / C(52, 5)

While not as common as some other hands, this probability is approximately 0.0014%. In a standard 5-card Poker game, you have about a 0.0014% chance of being dealt or forming a Straight Flush hand.

Probability of Royal Flush Hand

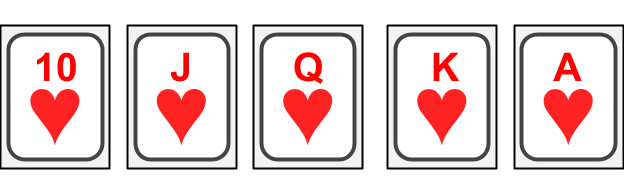
The “Royal Flush” hand is the rarest and most coveted hand. It consists of the Ace, King, Queen, Jack, and Ten in the same suit. 

Calculating the Probability of a Flush Hand:

• Total 5-card combinations (with 52 sample spaces):
• In the 52-card standard deck, there are C(52, 5) = 2,598,960 possible ways to select five cards, where C means “Combinations.”
• Number of Royal Flush Hands:
• There are 4 suits in a standard 52-card deck (Spades, Hearts, Clubs, and Diamonds), so there are 4 ways to attain a Royal Flush.

Now, let’s calculate the probability of getting a Royal Flush hand:

• Probability of a Royal Flush Hand in 5-Card Poker:

= (Number of Royal Flush Hands) / (Total 5-card combinations)

= (4) / C(52, 5)

This probability is extremely low and is approximately 0.00015%. In a standard 5-card Poker game, you have an infrequent chance of about 0.00015% of being dealt or forming a Royal Flush hand.  A Royal Flush is the pinnacle of Poker hands, and seeing one in a game is a remarkable and memorable occurrence.

Pre-Flop Probabilities

The pre-flop probabilities are the probabilities of getting a particular Poker hand before any dealt cards. The probabilities are the same for all players, regardless of their position at the table.

Understanding the probabilities before the flop (the first three community cards) in Texas Hold ’em Poker is crucial for strategic decisions. 

Starting Hand Probabilities:

Each player receives two private cards (hole cards), and the objective is to evaluate the likelihood of starting with solid hands.

Starting Hand Probabilities	Probability
Pair Probability	5.88%
High Card Probability	24.00%
Suited Cards Probability	23.53%
Connected Cards Probability	5.88%

• Pair Probability: The likelihood of being dealt a pair as hole cards is about 5.88%.
• High Card Probability: The chance of receiving at least one high card is approximately 24.00%.
  • (Ace, King, Queen, or Jack) in your hole cards.
• Suited Cards Probability: The probability of getting hole cards of the same suit is around 23.53%.
  • (e.g., two hearts, two spades).
• Connected Cards Probability: The chance of receiving consecutive hole cards in rank is approximately 5.88%.
  • (e.g., 8-9, 10-J).

Odds of Hitting a Hand on the Flop:

After the flop, you must calculate the odds of improving your hand based on the community cards.

Flop Improvement Probabilities	Probability
Hitting a Set (Three of a Kind) Probability	11.76%
Completing a Flush Probability	10.94%
Completing a Straight Probability	8.51%

• Hitting a Set (Three of a Kind) Probability: If you have a pair, the chance of getting a third card of the same rank on the flop is roughly 11.76%.
• Completing a Flush Probability: If you have two suited hole cards, the probability of getting two more of the same suit on the flop would be about 10.94%
• Completing a Straight Probability: If you have connected hole cards, the chance of getting two more cards on the flop to complete a straight is approximately 8.51%.

Post-Flop Probabilities

The probability of getting a particular Poker hand depends on the number of cards and the number of ways to get that hand. 

The pre-flop probabilities are the same for all players, regardless of their position at the table. The post-flop probabilities will vary depending on the cards on the board.

Improving Your Hand:

After the flop, you can calculate the odds of improving your hand based on the community and hole cards.

Post-Flop Probabilities	Probability
Hitting a Set (Three of a Kind)	8.26%
Completing a Flush	34.94%
Completing a Straight	32.36%

• Hitting a Set (Three of a Kind) on the Turn or River: If you have a pair after the flop, the probability of getting a third card of the same rank on either the turn or river is 8.26%.
• Completing a Flush on the Turn or River: If you have four suited cards after the flop, the probability of getting the fifth card of the same suit on either the turn or river is 34.94%.
• Completing a Straight on the Turn or River: If you have four consecutive cards after the flop, the probability of getting one of the missing cards on either the turn or river is 32.36%. 

Drawing Odds:

Understanding drawing odds is crucial for deciding whether to continue in the hand or fold.

Post-Flop Probabilities	Probability
Open-Ended Straight Draw	32.36%
Flush Draw	34.94%
Gutshot Straight Draw	10.77%

• Open-Ended Straight Draw: If you have four consecutive cards after the flop, the probability of completing the straight with either of the two missing cards on the turn or river is 32.36%. 
• Flush Draw: If you have four suited cards after the flop, the probability of completing the flush with the missing card on either the turn or river is 34.94%. 
• Gutshot Straight Draw: If you have an inside straight draw after the flop, the probability of completing the straight with one specific missing card on either the turn or river is 10.77%. 

Using Drawing Odds to Make Decisions

When deciding whether or not to call a bet with a draw, you should consider the drawing and pot odds. 

• You should call the bet if the drawing odds are better than or equal to the pot odds. 

For example, if you have a flush draw and the pot odds are 4:1, you should call the bet because the drawing odds (1 in 3) are better than the pot odds.

• You should fold the hand if the drawing odds are worse than the pot odds. 

For example, if you have a flush draw and the pot odds are 4:1, you should call the bet because the drawing odds (1 in 3) are better than the pot odds.

The Theory and Basic Rules of Poker Probabilities

In a Poker game, the likelihood of winning depends on various factors, such as the type of Poker game, the number of players at the table, and the skill level of each player. 

In the following section, learn about the basics of Poker, discover the different variants of the game, and explore their varying probabilities.

What is Probability in Poker?

Learning about Poker probability proves vital for strategic decision-making, enabling players to assess the chances of various outcomes and adapt their tactics accordingly. 

Developing Poker’s probability theory is crucial for high-stakes players who use math to assess pot odds and hand values.

Seven Types of Probabilities of Poker

Poker combines skill, strategy, and luck. The game goes through several betting rounds where players must make intelligent choices based on how likely they think certain card combinations will appear.

Understanding the Poker probabilities is essential for making optimal decisions and maximizing one’s chances of winning. Here, we will break down Poker possibilities into several key components.

Starting Hand Probabilities

It refers to the probability of being dealt specific Starting Hands. 

For example, the probability of being dealt pocket aces (two aces as hole cards) in Texas Hold’em is approximately 0.45% or 1 in 221 hands.

Flop, Turn, and River Probabilities

You determine these probabilities by calculating how likely your hand will improve as more community cards appear.

For example, the probability of flopping a flush draw (having two cards of the same suit with the potential to make a flush) is roughly 10.94% or about 1 in 9 hands.

Outs and Pot Odds

“Outs” are the cards that can help you complete your hand. 

In case of a drawn flush (9 outs) and the pot is $100, and your opponent bets $20, you have pot odds of 5:1 (you need to call $20 to win $120 potentially). If the odds of completing your flush exceed 5:1, it’s a profitable call.

Implied Odds

Implied odds consider potential future bets.

Let’s say you have a straight draw, and you estimate that if you hit, your opponent will pay you an additional $50 on the next street. This extra value is part of your implied odds calculation.

Expected Value (EV)

EV assesses the long-term profitability of a decision. 

For example, when you consider calling a $10 bet in hand with a 25% chance of winning a $50 pot, calculate your expected value (EV) like this: (0.25 * $50) – $10 = $2.50.

Bluffing Probabilities

Bluffing estimates the likelihood of your opponent folding in response to your bet. 

Suppose you’re bluffing with a weak hand and believe there’s a 70% chance your opponent will fold. In that case, you’re considering the bluff’s success probability.

Hand Ranges and Equity

Hand ranges involve considering all possible hands your opponent could have based on their actions. Equity calculations help you determine your chances of winning against these ranges. 

For example, if you hold a pair of eights (50% equity) against an opponent’s range, you win half of the time on average.

Probability Rules of Poker

Probability involves drawing a card or forming a hand in Poker. The basic rules of probability in Poker include concepts like:

The Deck

A regular deck has 52 cards, all with the same chance of getting picked.

Independence

Each card drawn is independent of previous draws. It means the odds of drawing a particular card remain constant with each new draw.

Combinations 

Probability often involves calculating the number of ways specific outcomes can occur. Combinations are essential for understanding the chances of forming different Poker hands.

The Basics of Poker

Playing Poker involves several key steps:

Setup

Gather a standard 52-card deck and chips for betting.

Deal 

The dealer mixes the cards and gives each player a certain number of cards, usually 2, facing down. These are your “hole cards.”

Betting Rounds

Players go one by one in a clockwise order and decide what to do based on how good their cards are. The main options are to “check” (passing the action), “bet” (putting chips in the pot), “call” (match a bet), “raise” (increase a bet), or “fold” (discard your hand and forfeit the round).

Community Cards

In Texas Hold’em and Omaha, additional community cards are dealt face-up in the center of the table. These are combined with your hole cards to form the best hand.

More Betting

Following the reveal of each round of community cards, another betting round takes place.

Showdown

When the final round of betting finishes, players still in the game show their cards, and the player with the best Poker hand gets the pot.

Winning Hands

Poker hands are ranked, with the royal flush being the highest and the high card the lowest. Common hand types include pairs, straights, flushes, and full houses.

Start Over

The dealer position rotates, and a new hand begins.

Five Common Variants of Poker

There are many Poker games, each varying in style, length, and complexity. From well-known classics to up-and-coming variations, these five types of Poker are worth a try.

• Texas Hold’em: The Poker Powerhouse

Gameplay:	Every player gets two hole cards, and five community cards are dealt face-up at different points in the game, known as the flop, turn, and river.  Players create their best five-card hand using their hole cards and community cards.
Probability (Average):	7.46% – This is the average chance of forming a winning hand.
Winning Odds (Average):	42% – On average, a player has a 42% chance of winning.

• Omaha: High Stakes and High Actions

Gameplay:	Each player gets four hole cards, and five community cards appear face-up during the game in stages. Players must use precisely three community cards and two-hole cards to create the best five-card hand.
Probability (Average):	8.15% – The average probability of forming a winning hand.
Winning Odds (Average):	35% – On average, a player has a 35% chance of winning.

• Seven-Card Stud: The Classic Poker Challenge

Gameplay:	Players start with two hole cards and receive five community cards one at a time. Players must use their hole cards and five community cards to make the best five-card hand.
Probability (Average):	11.84% – The average chance of forming a winning hand.
Winning Odds (Average):	23% – On average, a player has a 23% chance of winning.

• Five-Card Draw: Simplicity With a Dash of Deception

Gameplay:	Players receive five-hole cards and can swap up to three for new cards. They then make the best five-card hand using their hole and drawn c
Probability (Average):	8.06% – The average probability of forming a winning hand.
Winning Odds (Average):	38% – On average, a player has a 38% chance of winning.

• High-Low Chicago: Unique Twist of Poker

Gameplay:	High-Low Chicago splits the pot between the player with the highest and lowest hands. Players form the best high hand and the best low hand using their hole cards and community cards.
Probability (Average):	10.32% – The average chance of forming a winning hand.
Winning Odds (Average):	29% – On average, a player has a 29% chance of winning either the high or low pot.

Getting Acquainted With Poker Hand Terms

Poker Terms	Brief Definition
Probability	The likelihood of an event happening. In Poker, the probability is used to calculate the chances of winning a hand based on the cards dealt.
Odds	It is the ratio of the number of favorable outcomes to the number of unfavorable results.  Pot Odds – It is the ratio of the amount you need to call to the size of the pot. Pot odds determine whether you are getting a reasonable price to call a bet.
Poker Variants	There are many Poker variants, but some of the most popular include: Texas Hold’em – Texas Hold ’em is widely hailed as the most popular form of Poker worldwide. In this game, every player gets two hole cards facing down and five community cards revealed in three stages: the flop (three cards), the turn (one card), and the river (one card), the turn (one card), and the river (one card). Players can bet on their hands after each stage of the deal. The winner is the player with the best five-card hand, combining two-hole cards and five community cards. Omaha – Like Texas Hold’em, each player is dealt four-hole cards instead of two. Players must use two cards from their hand and three from the community to form the best five-card hand. Seven-Card Stud – Deals seven cards to each player, one at a time, facing downwards. After each card, players can bet. The player with the best five-card hand wins, regardless of their seven-card combination. Five-Card Draw – Each player starts with five face-down cards, can exchange up to three for new ones from the deck, and then place bets. The player with the best five-card hand wins. After that, they can place bets on their hands. The person with the best five-card hand wins the game.
Hands	A Poker hand is a combination of five cards. There are many types of Poker hands, but some of the most common include: High Card – A hand that doesn’t form any different specific Poker hand ranking; it’s valued by the highest card in the hand. Pair – The hand with two cards of the same rank. Two pairs – A hand with two pairs of cards of the same rank. Three-of-a-kind – A hand with three cards of the same rank. Straight – It refers to a hand in which the cards are in sequence, regardless of their suit. Flush – Set of five cards with the same suit, without considering their ranks. Full house – A hand with Three-of-a-kind and a pair. Four-of-a-kind – The hand with four cards of the same rank. Straight flush – A hand of five cards in sequential order and the same suit. Royal flush – It is a Poker hand containing cards ranging from ten to ace.
Outs:	The number of cards left in the deck can improve a hand.
Standard Deck	A deck consists of 52 cards, with 13 cards in each of the four suits.
Backdoor	A backdoor draw is a draw you have to both the turn and the river.
Flop	The dealer deals with the initial three community cards.
Turn	The fourth community card dealt by the game dealer.
River	The dealer deals with the final community card, completing the set of five.
Bad Beat	A bad beat is a situation where you lose a hand despite having a strong hand.
Buy-in	The buy-in is how much you must pay to enter a Poker game.
Pot	The pot is the amount of money wagered by the players in a Poker hand.
Ante	An ante is a mandatory bet all players make before dealing with the cards.
Action	Action refers to the betting and raising in a Poker hand.
Blinds	The blinds are mandatory bets made by the players sitting to the left of the dealer button. The small blind is usually half the size of the big blind.
Betting	The process of wagering money on the strength of your hand: Check – To check is to pass the action to the next player without betting. You can only check if you have yet to bet. Call – To call is to match the previous bet. Raise – To raise is to bet more than the previous bet. Fold – To fold is to give up your hand and forfeit your interest in the pot. All-in – To go all-in is to bet all your remaining chips.

The Frequencies Behind Poker Hands

Players benefit from understanding these frequencies as they give a clear picture of the chances for each hand, helping them make more strategic choices during the game.

This section will explain the frequencies behind various Poker hands, including standard 5-card and 7-card hands and lowball variations for both 5-card and 7-card Poker.

5-Card Poker Hands

Knowing how likely it is to get certain card combinations can help you make more brilliant moves in Poker. This table shows how often different types of hands appear in a game of 5-card Poker.

Hand	Frequency	Description
High Card	0.42%	This hand happens when you don’t have any special combination of cards, and the highest card in your hand is what counts.
One Pair	4.75%	This hand happens when you have two cards of the same rank and different other three.
Two Pair	0.48%	You have two sets of pairs with matching ranks.
Three-of-a-Kind	0.21%	You have three cards of the same rank and two others that don’t match.
Straight	0.39%	You have five consecutive cards, but they must have different suits.
Flush	0.19%	All five cards are the same suit, but they don’t have to be in order.
Full House	0.14%	You have three cards of one rank and two cards of another.
Four of a Kind	0.024%	You have four cards of the same rank and one other card.
Straight Flush	0.0014%	You have five consecutive cards in the same suit.
Royal Flush	0.00015%	It is the rarest and best hand, consisting of the Ace, King, Queen, Jack, and Ten in the same suit.

This table tells you how often you can expect to get different types of hands in a game of 5-card Poker. It uses percentages to show how likely each type of hand is.

Knowing how often these hands happen can help Poker players make better decisions during the game of 5-Poker hands.

7-Card Poker Hands

The complexity and additional cards in seven-card Poker make it a more challenging variant than five-card Poker, requiring players to possess greater hand analysis and strategic decision-making skills.

This table shows how often various types of hands appear in a 7-card Poker game like Texas Hold’em or Seven-Card Stud.

Hand	Frequency	Description
High Card	0.029%	This hand occurs when you don’t have any special combination of cards and the highest card in your hand counts.
One Pair	4.45%	You have two cards of the same rank, and the other five differ.
Two Pair	1.08%	You have two sets of pairs with matching ranks.
Three-of-a-Kind	0.96%	You have three cards of the same rank and four others that don’t match.
Straight	1.49%	You have five consecutive cards, but they must have different suits.
Flush	5.08%	All seven cards are the same suit, but they don’t have to be in order.
Full House	0.41%	You have three cards of one rank and two cards of another.
Four of a Kind	0.026%	You have four cards of the same rank and three others.
Straight Flush	0.015%	You have five consecutive cards that are all the same suit.
Royal Flush	0.00016%	This hand is the rarest and best hand, consisting of the Ace, the King, Queen, Jack, Ten, Nine, and Eight, all in the same suit.

Like in the 5-card game, these percentages tell you how likely each type of hand is.

Knowing how likely these hands are in games like Texas Hold’em or Seven-Card Stud can help you decide when to bet, fold, or raise. 

For example, flushes are more common in a 7-card game, so you should be cautious if your opponent shows signs of having one.

5-Card Lowball Poker Hands

In lowball Poker, the objective is to have the lowest possible hand. This table displays the frequency of different low-ranking hands in a 5-card lowball Poker game.

Hand	Frequency	Description
High Card	95.4%	In lowball, having a high card is the most common hand. It means you don’t have any pairs, and the card values are as high as possible.
One Pair	4.3%	A lowball one-pair hand means you have two cards of the same rank, but the rest are low, helping keep your hand relatively weak.
Two Pair	0.25%	This hand is an even rarer lowball hand with two sets of pairs, but the pairs consist of low-ranking cards.
Three-of-a-Kind	0.05%	Having three cards of the same rank in lowball is quite rare, and it still needs to be done with low-value cards.

These percentages help you understand how often you have a low hand.

The goal is to avoid high cards and aim for the weakest possible hand to win. So, having more high cards is a disadvantage in this game.

7-Card Lowball Poker Hands

Lowball Poker takes an exciting twist in a 7-card game like 7-card Razz, where the goal is to have the lowest possible hand. 

In this table, we’ll explore how often various low-ranking hands occur, shedding light on the challenges and opportunities in this unique Poker variant.

Hand	Frequency	Description
High Card	93.2%	In a 7-card lowball game, having high cards is the most common outcome. It means you have no pairs or matching cards, and the values of your cards are relatively high.
One Pair	6.1%	A one-pair hand is less common but occurs reasonably frequently in lowball Poker. It involves having two cards of the same rank while the rest are low-ranked.
Two Pair	0.5%	Two pairs in a lowball game are rare and typically consist of low-value cards, making this hand moderately stronger than high cards.
Three-of-a-Kind	0.2%	Obtaining three cards of the same rank in a 7-card lowball game is unusual. When it does happen, they are usually low-value cards.

In games like 7-Card Razz, understanding these frequencies helps players gauge the strength of their hands and make strategic decisions. 

The key is avoiding high cards and aiming for the weakest possible hand to secure victory, which can be challenging with the randomness of seven-card draws.

Difference Between “Probabilities” and “Odds” In Poker

Poker odds and Poker probability are related concepts players use to make informed decisions at the Poker table. 

However, they are different, and understanding their differences is crucial for effective Poker strategy.

Poker Probability

Probability refers to the likelihood of a specific event or outcome occurring in a hand or game. It deals with the theoretical chances of different hands or scenarios based on the total number of possible outcomes.

Poker probability is expressed as a percentage or a ratio, and it helps players understand the inherent likelihood of their situation.

For example, if you want to calculate the probability of being dealt a pocket pair (two cards of the same rank) in Texas Hold’em, you would consider that there are 13 ranks of cards, and for each rank, there are four suits. 
So, there are 13 * 6 = 78 possible ways to receive a pocket pair. The probability of getting a pocket pair is then 78/1326 ≈ 5.88%.

Probabilities help make informed decisions during a hand, such as calculating the odds of improving your hand or assessing the strength of your starting hand.

Poker Odds

Poker odds express the relationship between the number of favorable outcomes (winning) and unfavorable outcomes (losing) in a specific Poker hand or situation.

Odds are typically expressed as a ratio, telling you how much you can expect to win relative to your bet if you make a particular play.

For example, if you’re playing in a hand of Texas Hold’em, and you have a flush draw (four cards of the same suit), nine cards of that suit remain in the deck.

Your odds of completing your flush on the next card are 9 to 47 (9 favorable outcomes out of 47 possible remaining cards). You can express this as 9/47 odds.

The odds are valuable when deciding whether to call a bet, as they allow you to compare the potential payout (odds received) to the cost of the bet (odds against).

If the odds in favor of completing your draw are more significant than the pot odds, it may be a profitable call.

Poker Probability vs. Poker Odds: What Sets Them Apart?

The key difference between Poker odds and Poker probability lies in their purpose in the game is and what they convey to the player:

Poker Probability

• Poker Probability is all about determining the chances of a specific event occurring over time.

This aspect of Poker assists in making informed decisions about hand strength, on which starting hand to choose and comprehending the basic odds of various situations. Probability remains constant and does not alter within a single hand.

Poker Odds

• By analyzing the odds, one can determine whether a bet or call is advantageous based on the current circumstances.

Calculating Poker odds depends on the current situation at hand. These odds indicate the potential return on investment for a particular play, considering the cards needed versus the cards remaining in the deck. 

Example Scenario:

Let’s say you’re playing Texas Hold’em and have two spades in your hand (e.g., 9♠ and 7♠). 

On the flop, two more spades are revealed (5♠, Q♠, 2♦). You want to calculate your odds of completing a flush on the turn (the next card).

• There are 9 spades left in the deck (13 total spades – 2 in your hand – 2 on the flop).
• There are 47 cards left in the deck (52 total cards – 2 in your hand – 3 on the flop).

So, your odds of completing the flush on the turn are 9 to 47. In probability, you have roughly a 19.15% chance of hitting a spade on the turn.

However, your odds could change on the river, depending on whether you hit the flush on the turn. This dynamic use of odds guides your decision-making in the current hand.

Bottomline

A firm grasp on Poker probabilities and odds can significantly improve your ability to adjust your strategies during gameplay and give you realistic expectations for potential outcomes.

While a royal flush is considered the best possible hand, it is also the rarest. As you strive for higher-ranked hands, the likelihood of receiving them decreases significantly.

The key to winning in Poker involves understanding the mathematics behind the game. Always play your cards right, don’t just rely on the luck of the draw.